Nothing’s Lost When “Starting Over a Mortgage”

February 28, 2022

Planning & Saving, Real Estate

Eli Minzer was 10 years into a 30-year mortgage, and starting the clock back to month one seemed crazy to him. He had 240 payments left on his original $507,500 mortgage, which he’d taken out at 4%. A mortgage broker kept suggesting that Eli refinance the $400,000 he still owed to grab the 3% rate now available.

But it didn’t add up, so Eli suspected the broker was either uneducated or unethical. Everyone knew that earlier payments of a mortgage have much more interest embedded in them versus the later payments; starting the payment clock over didn’t make sense. He also had no desire to pay an additional 10 years’ worth of payments even if the rate on all of them was lower.

How does refinancing a mortgage ever make sense?

Mortgage Math

Mortgage math is confusing, and even large real estate investors often don’t understand it fully. But switching a long-term mortgage to a significantly lower interest rate is almost always a good idea. You can just accept that as a matter of fact, or you can run the relevant numbers. But it’s actually not hard to explain the math basics behind loan amortization and prove that there is no penalty for “starting a mortgage over,” so here goes. (Amortization means the calculation for how a loan’s principal is paid down over time.)

A Simple Example

To do so, let’s use a very simplified comparative scenario. Say someone takes a one-year loan for $100,000 at 4% and is offered two different repayment options: The first choice is to use a traditional full amortization schedule with 12 equal monthly payments of $8,515. The second, interest-only option includes monthly payments of just the $333 of interest that will accrue that month ($100,000 x [4%/12] = $333 ), with the entire $100,000 balance due only at the end of the year. (The interest-only option is not uncommon for commercial mortgages, by the way.)

See the attached figure.

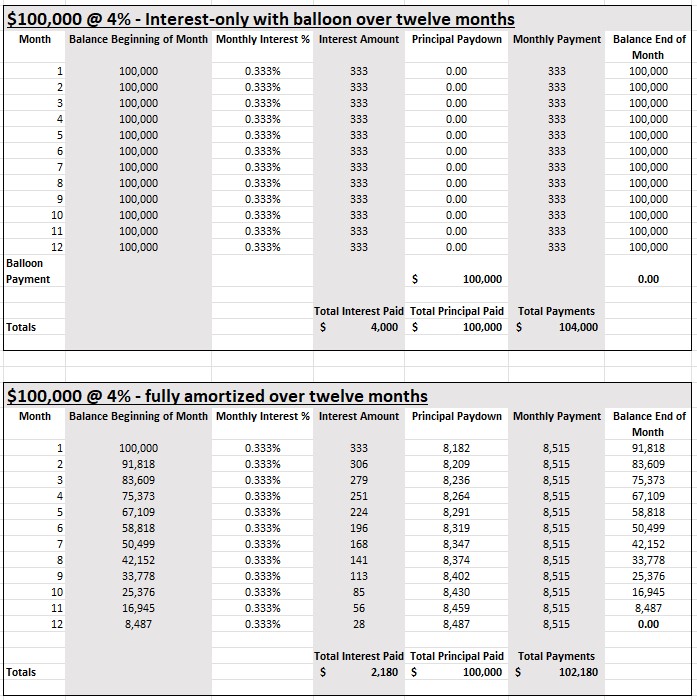
Interest Only

The math contained in the interest-only option is quite simple: One year of interest charged at 4% on $100,000 is $4,000. This is paid out monthly as $333 because 4% divided by 12 is .333%. Multiplying the $100,000 by .333% is how the bank comes up with the 12 monthly payments. Since there is no ongoing amortization (i.e., principal paydown each month), the principal balance remains constant at $100,000, as does the $333 charged monthly. The 12 $333 monthly payments indeed add up to $4,000, for an annual 4% interest rate.

Amoritzation

The amortization option is more nuanced. While the 12 payments are all $8,515, the breakdown in that number between interest and principal changes every month. But note this crucial factor: the rate being charged monthly stays the same—.333%, or 4% annualized; in month 1 this equals 333 ($100,000 x .333%). The reason you pay less interest in the second month is because by that time, the principal owed has gone down. Each payment multiplies the amount owed by the same .333% (i.e., 4% annually), but since some principal is also paid each month, the amount owed goes down and the interest charge for that months falls accordingly.

So, in an amortizing loan, the reason the dollar amount owed monthly falls month after month is because the amount owed falls month after month. The percent charged, though, is exactly the same for each payment. There’s no yichus or ma’alah to month 12 versus month one… except that by month 12 there’s almost no principal left to be repaid, so the interest charged that month is minimal even though the rate the balance is multiplied by remains identical—1/12 of 4%. (For month 2, since the amount owed then is down to $91,818, the interest amount charged for the month is lowered to $306 ($91,818 x.333%) and so on.)

See What You Save

This basic math also explains why the total interest paid on the amortizing loan is less than $4,000, or 4%, of the $100,000 originally borrowed. The 12-month interest-only option costs the borrower exactly $4,000 because the entire loan is outstanding for the entire year. But in the amortization option, the bank gets back a chunk of its money each month. Since the loan balance is a lot less than $100,000 for a full 12 months, the actual interest amount charged is also a lot less than $4,000.

Back to Mr. Minzer

Now, let’s get back to Mr. Minzer. He still owes the bank $400,000 and is scheduled to repay that in 240 equal amortizing payments charged at an annualized 4%. How would those 240 payments compare to those of a buddy who takes out a brand-new 20-year $400,000 fully amortizing loan at 4%? Would they be higher or lower, and what would the breakdown be between principal and interest?

This is a trick question, and if you’ve absorbed the earlier explanation, you aren’t reaching for your loan calculator. Mathematically and practically, those two sets of 240 payments (numbers 121–360 vs. 1–240) are identical.

A Lower Rate Equals Less Interest

A loan doesn’t know or care whether you’ve “put in your time.” Payment number 121 of Mr. Minzer’s current 4% loan is $2,423, which breaks down to $1,332 in interest ($400,000 x [4%/12] and $1,090 of principal repayment. And payment number one of a 20-year, $400,000 fully amortizing loan at 4% is exactly the same. To calculate monthly interest, one simply multiplies the amount owed by the interest rate divided by 12.

Therefore, lowering the rate charged against the outstanding $400,000 from 4% to 3%, as the mortgage broker proposes, is a no-brainer. By refinancing his $400,000 outstanding balance to a new fully amortizing 20-year loan at 3%, the monthly payment falls from $2,423 to $2,218. The interest charged the first month will be $1,000 instead of $1,332. And this interest savings will total tens of thousands over the remaining life of the loan. And the calculation is clear to us now: $400,000 is multiplied by 1/12 of 3% instead of by 1/12 of 4%. Assuming a similar 20-year repayment rate, a lower rate charged on the same $400,000 equals less interest. Guaranteed.

Lowering the Rate Versus Extending the Loan

To end, Mr. Minzer may still be confused because the broker is also proposing to extend the loan’s life by 10 years. If he refinances into a new 30-year mortgage with 360 payments (instead of 240), his monthly payment will go down much more drastically, from the original $2,423 to just $1,686; the interest charged in month one will still be $1,000 ($400,000 [3%/12]) versus the original $1,332 ($400,000 [4%/12]), but his overall interest for the life of the loan will rise a bit because he’s holding onto the bank’s money for much longer. Taking the longer loan option may or may not be a good idea, but, as we illustrated here, lowering the interest rate definitely is.